• Students will be able to state, proof, and use the theorem that states that the sum of any two lengths of any triangle is greater than the length of the third side.

Expected prior knowledge:

• Basic triangle properties
• Theorem that states two sides of a triangle are equal if and only if their opposite angles are equal
• Theorem that states if two angles of a triangle are unequal, then the sides opposite are unequal in the same order
• Law of cosines
   

Anticipatory Set:

• Give each of the students a set of three segments (that cannot be formed into a triangle; i.e., the sum of two of the sides is not greater than the third side) and ask them to form a triangle using their the 3 segments they were given.
  • Students see that not every set of three segments can form a triangle.
  • Gives students motivation to figure out what must be true about three segments to form a triangle.
     

Instruction:

• Give students each a “hinge” (see below) with two fixed lengths of triangle sides and a ruler to form the third side.
  • Students will find the smallest and largest possibilities for the third side of the triangle and write their results in an organized chart on the board.
  • Students then look at all the results on the board and analyze the results and make conjectures about sides of a triangle.

    Hinge:

    Students are given a hinge with two fixed sides and a ruler to use for the third side of a triangle. The hinge is a strip cut out of card stock and folded for the “hinge”. Each student is given a “hinge with different length sides.
    Example of hinge:

  
  
   

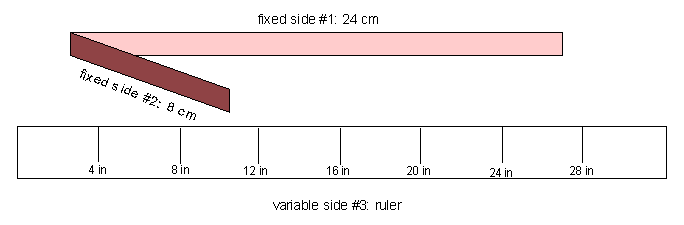
• Discuss the findings and the conjecture
• Walk through as a class the outline of a formal proof to show our conjecture is always true and not just for the examples we tried in class.
  • View proof outline here. The proof outline was created using Math Composer.
  • Students will write up a formal proof for homework.
• Optional: Show students another way to prove the same theorem. As a class, go through how you could use the law of cosines in a proof.
• Make up a worksheet for homework with further problems relating to this theorem.